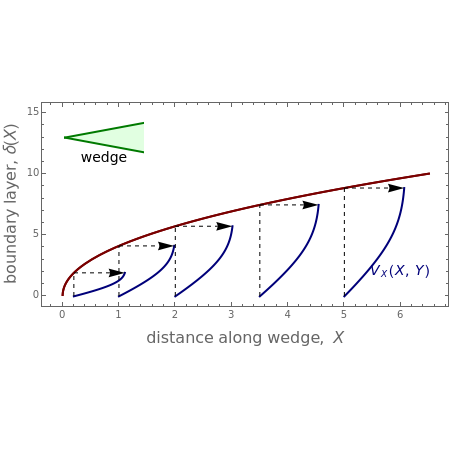
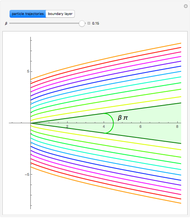
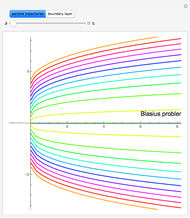
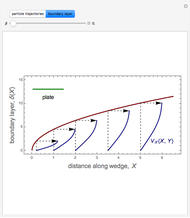
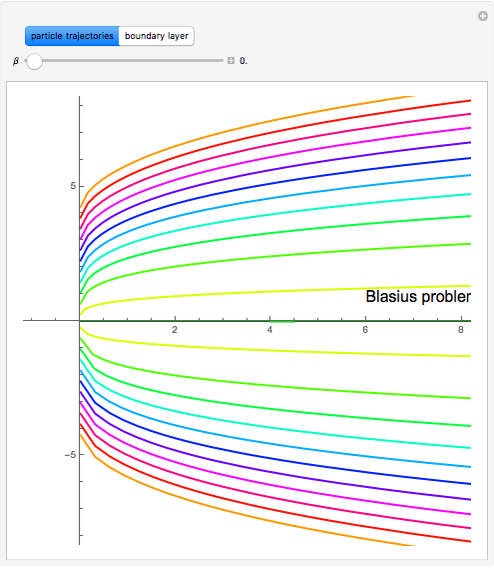
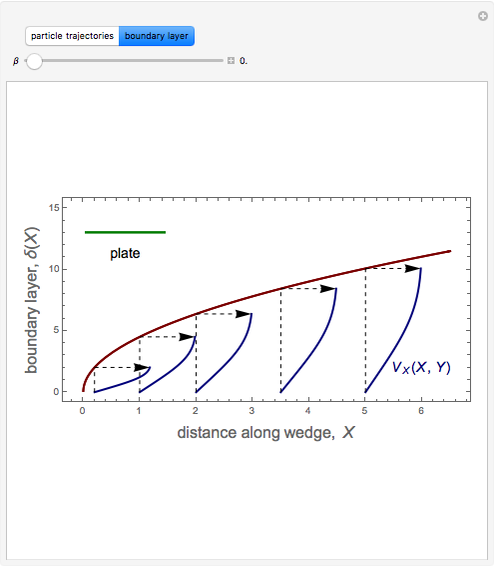
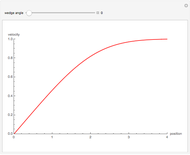
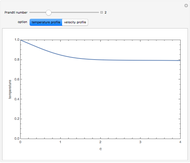
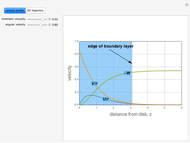
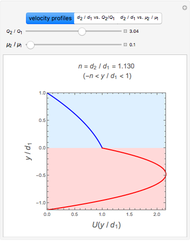
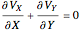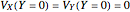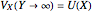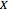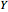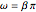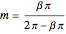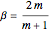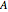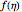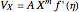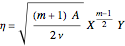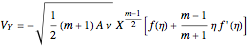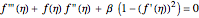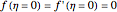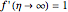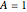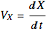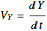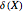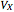Boundary-Layer Flow Past a Semi-Infinite Wedge: The Falkner-Skan Problem

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
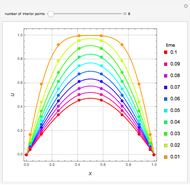
In 1904 Prandtl showed that the effects of viscosity at a high Reynolds number could be represented by approximating the Navier–Stokes equations with the celebrated boundary-layer equations, which for two-dimensional steady flow reduce to:
[more]
Contributed by: Housam Binous and Brian G. Higgins (June 2011)
Open content licensed under CC BY-NC-SA
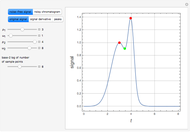
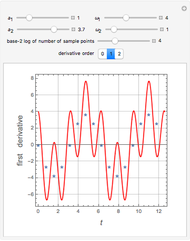
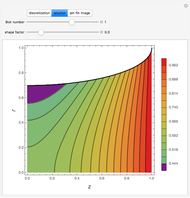
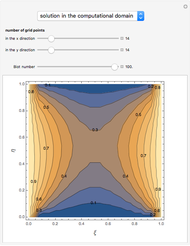
Snapshots
Details
References
[1] L. G. Leal, Advanced Transport Phenomena: Fluid Mechanics and Convective Transport, Cambridge: Cambridge University Press, 2007.
[2] A. D. Polyanin, A. M. Kutepov, A. V. Vyazmin, and D. A. Kazenin, Hydrodynamics, Mass and Heat Transfer in Chemical Engineering, Boca Raton, FL: CRC Press, 2002.
Permanent Citation