Magnet Types in Particle Accelerators

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
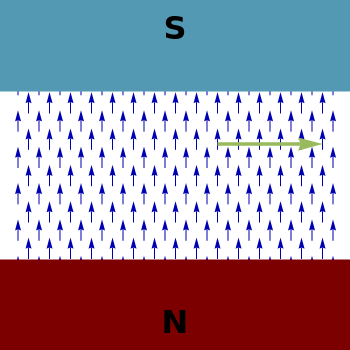
Major components of circular particle accelerators (for example LHC in CERN) are its magnets. In this Demonstration, you can study the forces on moving charged particles in a magnetic field.
[more]
Contributed by: Jakub Serych (March 2010)
Open content licensed under CC BY-NC-SA
Snapshots
Details
The magnetic force (Lorentz force) on a particle with charge  in a magnetic field of strength B moving with velocity v is:
in a magnetic field of strength B moving with velocity v is:
F=  v × B.
v × B.
This force is centripetal, directing the particle into a circular path. This is the role of the main dipole magnets.
Quadrupole magnets can focus the beam of particles, but only in one direction. They focus the charged particles in the vertical direction, but unfortunately also defocus them in the horizontal direction. It is possible to focus particles back into the horizontal direction with a quadrupole magnet rotated by 90 degrees. The optimal result is obtained by alternating these two orientations.
The strength of the magnetic field along the focusing axis of a quadrupole grows linearly from the center of the field, where it is zero. There are many pairs of focusing-defocusing magnets (so called FODO cells) in circular accelerators.
Sextupoles can be used in combination with quadrupoles to align the particle momentum of particles as the radial positions of the circulating particles change with momentum. The strength of the field of sextupoles along the horizontal axis grows quadratically from the center, where it is zero.
References:
[1] N. Marks, "Conventional Magnets for Accelerators," The Cockcroft Institute.
[2] S. Baird, "Accelerators for Pedestrians," European Organization for Nuclear Research.
Permanent Citation