Method of Integer Measures

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
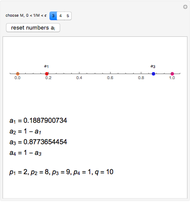
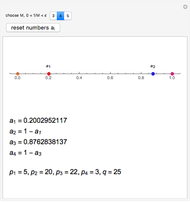
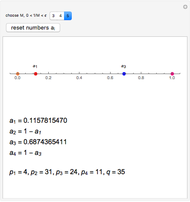
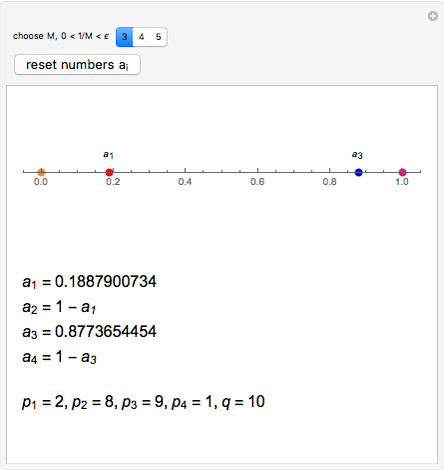
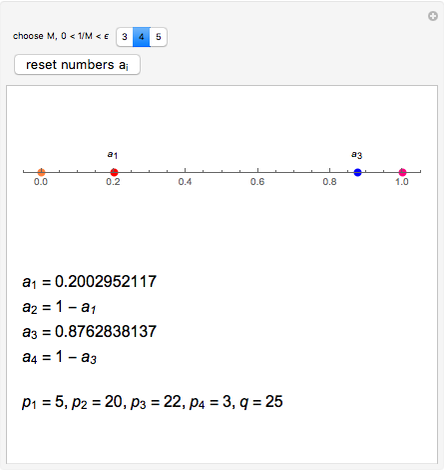
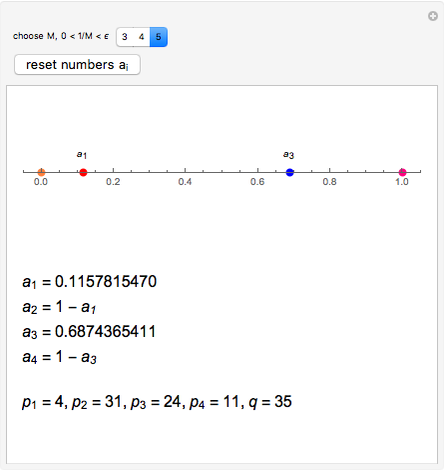
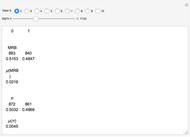
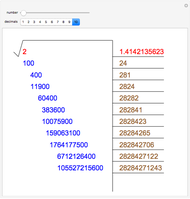
This Demonstration illustrates Benko's idea of "integer measures": Given positive real numbers  , it is always possible to find integer weights
, it is always possible to find integer weights  such that whenever
such that whenever  for two subsets
for two subsets 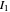 and
and 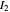 of
of  , then
, then 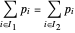 . This claim is a consequence of the fact that the numbers
. This claim is a consequence of the fact that the numbers  can be approximated by rational numbers
can be approximated by rational numbers 
 with any given uniform accuracy. Here
with any given uniform accuracy. Here 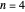 ,
, 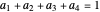 , and the top control can be used to set the accuracy to 1/3, 1/4, or 1/5.
, and the top control can be used to set the accuracy to 1/3, 1/4, or 1/5.
Contributed by: Mateja Budin and Izidor Hafner (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
The method is proved by following lemma:
Lemma 1. Let  be real numbers. For each
be real numbers. For each 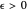 it is possible to approximate
it is possible to approximate  simultaneously by rational numbers
simultaneously by rational numbers  , in the sense that
, in the sense that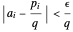 (
(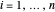 ). In addition, if all the
). In addition, if all the 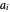 are positive, then the
are positive, then the 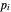 can be chosen to be positive, where
can be chosen to be positive, where  is replaced with
is replaced with 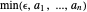 .
Proof. Let
.
Proof. Let 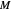 be a positive integer satisfying
be a positive integer satisfying  . Then by the pigeonhole principle, among the
. Then by the pigeonhole principle, among the 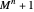 points
points  (
( ), where
), where 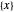 denotes the fractional part of
denotes the fractional part of 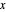 , there are at least two numbers
, there are at least two numbers 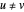 such that
such that 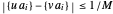 for all
for all 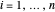 . Then
. Then 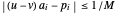 for some integers
for some integers 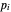 . The statement is proved if we put
. The statement is proved if we put 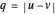 .
This lemma was used in the elementary proof of Hilbert's third problem.
.
This lemma was used in the elementary proof of Hilbert's third problem.
Reference
[1] D. Benko, "A New Approach to Hilbert's Third Problem," American Mathematical Monthly, 114(8), 2007 pp. 665–676.
Permanent Citation