Details

Let be a polyhedron with triangular faces and vertex set . An infinitesimal isometric deformation of is a map such that

at

for all edges of [3, definition 2.1].

This condition is equivalent to .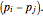

A polyhedron is infinitesimally flexible if it has an infinitesimal isometric deformation in which some of its dihedral angles change.

A theorem of Blaschke and Liebmann states: Let be a polyhedron combinatorially isomorphic to the octahedron. Color the faces of black and white so that each pair of adjacent faces has different colors. Then is infinitesimally flexible if and only if the four black faces intersect at a point, or equivalently, if the four white faces intersect at a point. The intersection points can lie at infinity [3, theorem 2.8].

The polyhedron is the simplest nonconvex polyhedron that cannot be triangulated into tetrahedra without adding new vertices [4].

References

[1] P. R. Cromwell, Polyhedra, Cambridge: Cambridge University Press, 1997 pp. 222–223.

[2] M. Goldberg, "Unstable Polyhedral Structures," Mathematics Magazine, 51, 1978 pp. 165–170.

[3] I. Izmestiev, "Examples of infinitesimally Flexible 3-dimensional Hyperbolic Cone-Manifolds," Journal of the Mathematical Society of Japan, 63(2), 2011 pp. 363–713. projecteuclid.org/euclid.jmsj/1303737798.

[4] Wikipedia. "Schönhardt polyhedron." (May 27, 2016) en.wikipedia.org/wiki/Sch%C3%B6nhardt_polyhedron.