Details

Consider a rigid toroidal indenter with principal radii and , , 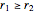pressing against an elastic semi-infinite body of elastic modulus (Young's modulus) and yield strength with a force only along the axis, and without friction. Let the major and minor radius of the contact ellipse be and , respectively. The eccentricity of the contact ellipse is independent of the load [1, 2] and is given by the solution to the following equation. Note that when , the contact patch is circular: and .

.

Here and are the complete elliptic integral functions. Let . For given , we can find the geometric mean radius , penetration and maximum pressure using formulas in Johnson [1]. We can calculate and .

,

,

.

As the load is increased, at a certain point along the axis and under the indenter, the material will yield. Since the shear stress components in the coordinate system chosen vanish by symmetry, the normal stresses become the principal stresses. Then, the location of initial yield can be predicted using one of three common criteria: maximum reduced stress, shear stress or von Mises stress.

The formulas for the normal stresses along the axis are given in [1]. In the following, and over-bar represents values normalized by , for example, . When , the formulas for the normal (principal) stresses , , are tedious to calculate [1, 2].

,

,

,

,

,

,

,

,

,

,

,

.

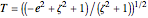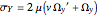When the toroidal indenter becomes spherical, the normal (principal) stress components , and are given by

,

.

To facilitate comparison with given yield strength of the material, we can write the following formulas.

where ,

,

.

Writing , the three failure criteria can be written as: reduced stress (), shear stress ) and von Mises stress ). Solving for the location of initial yield below the indenter using the Mathematica function FindMaximum, we obtain from .

This Demonstration codes this formulation allowing you to see the relative dimensions of the indenter and the contact ellipse when zooming in. It also reports the values at yield for given material properties , and for the criterion chosen.

Two plots are shown to the left. Top shows the variation in contact dimensions , and with increasing load .

The bottom shows the variation in penetration (red curve, right axis) and position (blue curve, left axis) with load . Dotted lines plot the position at yield for the criterion chosen.

A key fact is that is very sensitive to and . The earlier equation can be rewritten as

.

The equation above has two roots; one of which is for any . This is the correct root to use when or .

References

[1] K. L. Johnson, Contact Mechanics, 1st ed., Cambridge: Cambridge University Press, 1985.

[2] H. R. Thomas and V. A. Hoersch, Stress Due to the Pressure of One Elastic Solid upon Another, Bulletin No. 212, Engineering Experimental Station, University of Illinois, 1930.